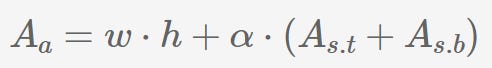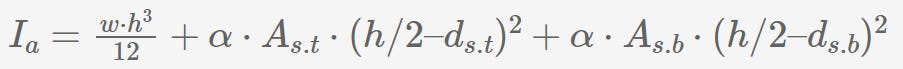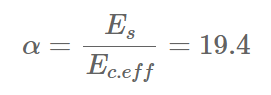The Moment Of Inertia Of Uncracked Reinforced Concrete Sections
And other composite sections
Happy Wednesday, 👋👋
The last 2 weeks, you got an introduction to what the moment of inertia and section modulus are and how you calculate them for “easy” and common cross-sections.
BUT, we didn’t cover one of the most used cross-sections in structural engineering, the reinforced concrete cross-section, which is a composite section.
So, today, we’ll cover how you calculate the moment of inertia of uncracked reinforced concrete sections, because you need that when you calculate a reinforced concrete beam or floor. This can also be applied to other composite sections like timber and concrete floors or a section with steel beams and a concrete deck (used in bridges).
Reinforced concrete sections are mostly cracked. In that case, the moment of inertia is a lot smaller. We’ll look at this scenario next week.
So, today we’ll cover:
Why are the moment of inertia’s of composite sections different?
The calculation process
A calculation example
So let’s get into it. 🚀🚀
Why Are The Moment Of Inertia’s Of Composite Sections Different?
Because a composite element consists of 2 materials which most likely have different stiffness and strength parameters.
Imagine this: Take a few pieces of paper and bend it. It’s pretty easy, right? This is because the bending stiffness of the paper is very low (E-modulus * moment of inertia).
Next, let’s add a pen in between the paper. Now it’s very difficult to bend it, right?
It’s because the pen has a much higher bending stiffness. This is an extreme example because the stiffnesses of paper and pen are so far apart from each other, but it’s the same principle for reinforced concrete sections (if we leave out cracking for a moment). Concrete has a lower E-modulus as steel (reinforcement), which means that a concrete beam without steel bends more easily.
To account for this increased stiffness with reinforcement in the design calculations like bending and deflection verifications, we calculate the moment of inertia of the composite section, taking into account the different E-moduli.
Alright, let’s have a look at how we calculate the moment of inertia. 👇👇
Calculation Process
Here are the steps, we need to follow to calculate the moment of inertia of a rectangular reinforced concrete section (we’ll calculate and define all parameters in the next section):
Define properties of the reinforced concrete section (Longitudinal reinforcement at bottom and top, E-moduli of steel and concrete, cross-sectional dimensions, etc.)
Ratio between moduli:
Cross-sectional area (composite rectangular section)
Moment of inertia (composite rectangular section)
Calculation Example
Now, let’s apply this to an example with the following parameters
Geometrical parameters
Material parameters
1. First, we’ll calculate the ratio between the reinforcement and concrete moduli:
There you can see that the reinforcement is 19.4 times “stiffer” if you use the same amount of material.
2. Next, we’ll calculate the cross-sectional area of the composite section, taking into account that the reinforcement is stiffer:
3. Then, as the last step, the moment of inertia of the composite section:
Conclusion
Now, the same principle can be applied to other composite cross-sections, like timber and concrete or steel and concrete sections. You calculate the ratio between the 2 moduli and apply this ratio to the moment of inertia of that material.
In most cases, concrete cracks. This happens when the tensile strength of the concrete is reached. Once concrete cracks, it loses a lot of its stiffness and all the tension forces of a cross-section are taken by the reinforcement. This moves the neutral axis of the cross-section and therefore also the moment of inertia.
Cracked cross-sections lead to much bigger deflections due to its reduced stiffness.
That’s why, next week, we’ll look at how to calculate the moment of inertia of cracked reinforced concrete sections.
See you next Wednesday. 🚀🚀
Have a great rest of the week and a great weekend. ✌️✌️
Cheers,
Laurin.
Enjoy the newsletter? Please forward to a friend you think could like our structural engineering content. It only takes 14 seconds. Making this one took a few hours. Just forward this link: https://www.structuralbasics.com/newsletter/